Ask Miss Science

Busy Life

I'd originally intended to answer questions about once every week or three.

But, that might have been a bit too optimistic.

Thanks to everyone (well, except for the spam) for the comments!

With luck, I will continue posting soon.

posted by Ask Miss Science @ 6:30 PM 1 comments

How To Make A Shih-Tzu Float

Dear Miss Science: Given a 16.5-lb. Shih-Tzu which breaks down to approximately 14.5 lbs. of dog and 2 lbs. hair, how many standard-size mylar balloons filled with helium would I have to tie to him to achieve gravitational lift-off, and how many would I have to use to achieve an aerial height of at least eighteen inches?

-- Helly (actually posting for Finnovar the cat, because of course I would never need to know such a thing)

Finnovar the Huge in his natural habitat:


Though this question seems relatively simple, the answer -- or, rather, its associated explanation -- is a bit lengthy. I opted for length so that I could explain how I arrived at the answer in some detail. However, I understand that there are those who will not be interested in the details. To help readers find the details that most interest them I have divided the answer into appropriate sections. The reader is encouraged to skim through and read only those portions of interest.

I would also like to thank Helly and Dad for proofreading an early version of this!

The Answer (vague though it may be)

I cannot give you a number that will work in all cases.  Neither can I tell you how to achieve the 18-inch hover without knowing very specific information about the particular air in which your dog will be floating.

However, I can give you an approximate number of balloons required simply for lift-off assuming that your air is at about 77° F and that the air pressure is 1 atmosphere. That answer is somewhere between one thousand and two thousand, depending on the nature of the balloons being used.  I got that number range from calculations based on measurements of the masses and volumes of two balloons I purchased.

Basic Principles

The calculation itself contains quite a few steps, but the basic principle isn't very hard to understand. In short, the combination of dog and balloons must be lighter than (weigh less than) the air that would otherwise be present (Figure 1).

Figure 1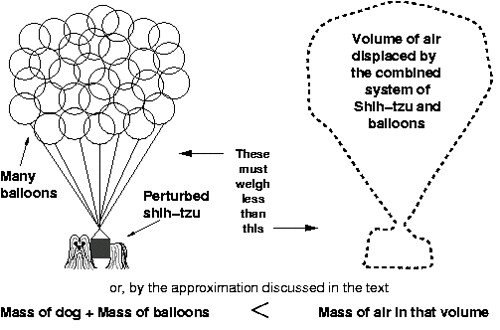


Put another way, the density of the dog and balloon system must be less than or equal to the density of the air surrounding them (recall that density is mass divided by volume).

Note that by using density I am using mass where, technically, I should use weight. However, for this problem the difference is trivial and mass makes the calculation simpler. The curious reader should see Note 1 for more information.

Here is the density sentence (just above, starts with "Put") written as an equation:

Density of air >= Density of dog and balloons     [Equation 1]

If the density of the dog and balloon system is lower than the surrounding air, the dog and balloon system will rise.  Since, typically, air becomes less dense as one rises, the dog and balloon system will continue to rise until a point where their density is the same as the surrounding air. 

In order to predict the number of balloons needed to achieve the 18-inch hover, you would need to know, in some detail, how the density of the air around the dog and balloons varies with height.  A far simpler approach would be to add and subtract balloons in-situ until the desired height is achieved.

However, before you start, it's a good idea to estimate the required number of balloons.  Based on my first calculation, you would need about 1400 balloons, give or take 250.  But, when I tried a second balloon, I calculated a slightly larger number. Given the differences between the two measurements, it might be safer just to say that you will need between one thousand and two thousand balloons. See the measurements section for more information about the two balloons I used.

Calculation Details:

Readers who already know how to perform this calculation might want to skip this section. It was written for readers who do not engage in these sorts of calculations regularly, and the calculations are explained in great detail. Readers who are not so experienced and wish to gain understanding of this sort of calculation are especially encouraged to read this section. If you are in this category, it might help to follow along with pen and paper, writing down the equations and notes regarding their meaning. Skip to the measurements section.

In this section, I'll explain how I got the base number of balloons (1400) for the first balloon. The calculation would be the same for any other balloon, but using the mass and volume of that balloon. At the end, I'll explain very briefly how I decided on the 1000-2000 range.

We'll start with Equation 1:

Density of air >= Density of dog and balloons     [Equation 1]

To make the math easier, let's only be concerned with the case where the two densities are equal. Doing this gives us the minimum number of balloons we need -- then we can add more balloons until the dog rises to the desired height.  So, I'll rewrite the equation:

Density of air = Density of dog and balloons     [Equation 2]

To find the number of balloons, we need to modify this equation so that it contains a number of balloons.  Once we've done that, we'll need to do a little algebra.  Then we'll have our number. 

Since the right side of the equation concerns the balloons, we should start by taking a closer look there. It's usually best to start by examining the definitions of any terms. We'll get to "dog" and "balloon" shortly. For now, though, let's define density.

Density is mass divided by volume.  As translated into mathematical notation, that is:

D = M / V      [Equation 3]

where D is density, M is mass and V is volume.

Now we need to apply Equation 3 to our situation.  Here, the density of our system is the mass of the whole system over the volume of the system.  In other words, we need to add up all the masses (dog, balloons, hair, harness, hair, string, hair, etc.) and divide that combined mass by the volume taken up by all those items.  Written mathematically, that is:

D_{dog and balloons} = (M_dog + M_{all balloons})  /  (V_dog + V_{all balloons})   [Equation 4]

In Equation 4, M_dog and V_dog refer to all dog-related masses and volumes (dog, hair, harness, collar, etc.), and M_{all balloons} and V_{all balloons} refer to the combined masses and volumes of all balloon-related items (string, mylar, helium, etc.). 

We can easily work a number of balloons into the right side of Equation 4 by assuming that the total mass of all the balloons can be determined, approximately, by multiplying the mass of one balloon by the number of balloons.  The volume of the balloons can be determined similarly.   (This assumption, of course, is a source of error in our calculation, but at least it gives us an answer.)  Now, we can say:

D_{dog and balloons} = (M_dog + N*M_balloon)  /  (V_dog + N*V_balloon)    [Equation 5]

Here N is the number of balloons, M_balloon is the mass of one balloon, and V_balloon is the volume of one balloon.  N is the number we want.  If we substitute the right side of Equation 5 for the right side of Equation 2, we get:

D_air =  (M_dog + N*M_balloon)  /  (V_dog + N*V_balloon)    [Equation 6]

Here, "D_air" = "Density of air" (for brevity).  After a little algebra, we get the result in Equation 7, below.  It is left as an exercise to the curious reader to check my algebra.

N = (M_dog - V_dog*D_air) / (V_balloon*D_air - M_balloon)   [Equation 7]

Now, we need to see if we have all the numbers we need.  If we don't, then we need to find some way to get them.  The question only supplied one number, the mass of the dog (M_dog).  We also need the volume of the dog (V_dog), the density of the air (D_air), and the mass and volume of a balloon (M_balloon and V_balloon). 

Truth be told, the volume of the dog can be ignored, but it would take a paragraph or two to explain why, so I'll leave it in.  I estimated that the volume of a shih tzu is about 10 liters, give or take 5.  That's a pretty reasonable estimate.  An average shoebox is about 5 liters, so 10 might even be a bit high, but it won't make much difference to the final answer.  So, we have a number:   V_dog = 10 L.

The density of air is available from a number of sources. I calculated the density of air at 1 atm pressure and a temperature of about 77 °F using information in this document (pdf file) concerning the composition of air. The density I calculated is 1.184 grams per liter. I'll spare you the details of how I calculated the density here. Feel free to ask, though, if you want to know.

I measured and/or calculated the other values.  Detailed descriptions of these procedures can be found below, but I'll give the answers here.  I measured the mass of the empty balloon-skin and its string as  11.13 g, and I calculated the mass of the helium in the balloon to be 2.65 g.  The combination of these masses gives M_balloon = 13.78 g.  I measured the volume of the filled balloon and found V_{filled-balloon}= 16.2 liters. 

I also converted the weight of the dog in pounds to mass in grams.  The mass in grams is 7484.2.

Now, we know all of the numbers we need and can substitute them in to Equation 7:

N = ( (7484.2 g) - (10 L)*(1.184 g/L) ) / ( (16.2 L)*(1.184 g/L) - (13.78 g) )   [Equation 7, substituted]

or,

N = 1383.57

Assuming we won't have any partial balloons, at least 1384 balloons will be needed.  However, considering my assumptions about the dog volume and the lack of data concerning the consistency of grocery-store balloons, it seems more reasonable to simply say that around 1400 balloons will be needed.  Once I figured in the error sources known to me, I found that the number might actually be somewhere between about 1150 and 1650.  I will spare you the details of this calculation, but I got the range by performing the calculation two more times, once assuming all the errors made the number as high as possible and again assuming all the errors made the number as low as possible.  After considering the second balloon (see below) and all the error sources not known to me, I decided it would be safest to say the number is somewhere between one thousand and two thousand.

Note that these numbers, as vague as they are, are only true for air at 1 atm and 77 °F. If your air is hotter or colder or a high- or low-pressure system is in the area, the numbers will change.

Other Measurements and Calculations:

Top. Basic Principles. Calculations.

In this section I describe how I measured the volumes and masses of the balloons and, briefly, how I calculated the mass of helium in the balloons.

General Notes Regarding the Measurements:

I looked around on the internet, but was unable to find any information on mass or volume of mylar balloons (except for one technical paper -- see Note 2).  So, I bought a balloon.  The first balloon I purchased wasn't mylar -- rather, it was a thin, non-elastic plastic without the metallic luster of mylar.  After deciding that I should answer the question as asked, I also bought a mylar balloon.   In both cases, I picked the most common size available at the large-chain grocery store from which I purchased the balloon.  Each cost $2.99 plus tax.  Although mylar is actually a form of plastic, I will use the term "mylar" to refer to the mylar balloon and "plastic" to refer to the other.

One should note that the procedures outlined below would not be acceptable for traditional, elastic balloons.  Since mylar and similar balloons are not elastic -- that is, they will not significantly compress the helium inside them -- the simple procedures outlined below are sufficient.

Measuring the Balloons' Volumes:

First, I measured the volumes of the filled balloons.  To do this, I filled a 10-gallon plastic storage bin completely full of water.  Since the mylar balloon had deflated slightly (I keep my house a bit cold), I used hot water for the mylar balloon so that the heat from the water would cause the balloon to fully expand.  After filling the bin with water, I pushed the balloon just barely under the surface making sure to keep as much of the balloon and as little of my fingers, as was possible, under water.  It was difficult to maintain a perfect balance, which will certainly introduce error into the measurement.  However, gauging the sizes of my fingertips and the small amount of balloon I left sticking out, I'd say the error will be on the order of a few milliliters (one-thousandth of a liter) and maybe less.   Pushing the balloon under water, of course, caused water to flow out of the bin.  I held the balloon still until water stopped flowing out of the bin.  Then, I removed the balloon and shook it so that most of the water on it would go back into the bin.

According to the principle usually associated with Archimedes (remember the "eureka!" story about the bathtub, the naked guy and the king's crown? -- I used the same idea here), the volume of water displaced from the bin is equal to the volume of the balloon.  To find that volume, I used a 1.50 liter bottle marked in 0.25 liter increments to refill the bin.  I kept up with the number of 1.50 liter amounts needed to refill the bin, which gave me the volume of the balloon.  The total volume I measured for the plastic balloon is 16.2 L and for the mylar balloon, 15.3 liters.  I estimate the error to be about 0.5 L and probably less.  See below in Note 2 for additional, somewhat technical discussion of this.

As an interesting side note, the small amount of water that would not shake from the balloon was enough to keep the balloon from floating.  Once I fully dried the balloon, it floated again just fine. 

As another interesting side note, since the plastic balloon appears to have been slightly larger (again, see Note 2), it would be more cost-effective to use the plastic balloon.

Measuring the Balloons' Masses:

Next, I needed to measure the mass of the balloon skin and string.  I couldn't measure the mass of the filled balloon because it would not have lain on the balance pan.  So, I punctured the balloon and massed it on an analytical balance (a very accurate and precise scale) where I teach.  Actually, I measured the mass on several balances and took the average value.   I left the string attached since, presumably, the string will be used to attach the balloon to the dog.  Using less string -- or a lighter string -- will somewhat lower the number of balloons that are required -- I measure the no-string mass, too, just to see if it would be different.

To get the mass of the helium in the balloon, I used the ideal gas law, PV=nRT.   This law allows us to calculate the number of atoms of a gas (n -- this is actually number of moles of gas, but the idea is similar) that are present at a certain pressure (P), temperature (T) and volume (V). The number of (moles of) atoms can easily be converted into a mass using the molar mass for helium. Helium's molar mass is the number just under the "He" on most periodic tables.

Notes:

Note 1: Weight is, technically, more appropriate than mass because weight includes the effect of gravity. If the somewhat significant gravitational field of the Earth were not present, this question would be rendered quite meaningless. However, since the size of the shih tzu and balloons system is very small compared to the diameter of the Earth, it is not important to account for variations in the effects of gravity. If, however, we were discussing a shih tzu, for example, tethered via a lengthy cable to a satellite, then we might do well to use weight instead of mass. This, of course, would also complicate the mathematics considerably. Back to reference in text.

Note 2: As an aside, I found, on the internet, the text of a paper published in the Journal of Nonlinear Mathematical Physics (Volume 11 Supplement, pp. 55-65, 2004).  The paper treats the geometry of a mylar balloon using elliptical integrals and reports that the volume of a mylar balloon with a deflated diameter of 9 inches to be 15.18 L.  Since I still had the mylar balloon around, I measured its deflated diameter and found it to be 9 inches.  The excellent agreement between these results indicates that my volume measurements were pretty good.  Unfortunately, I no longer had the other balloon available.  If its diameter were only slightly larger, say 9.25", then the volume would have been about 16.2 L.  My measurements will necessarily be larger than the calculations for two reasons.  One, I was unable to shake off all of the water clinging to the balloons.  Two, each balloon contains a small additional volume at the base where the balloon is filled. But, neither of these amounts constitutes more than a few dozen milliliters. The largest discrepancy is most likely due to inaccuracies in the equipment I used. Back to first reference to Note 2.

posted by Ask Miss Science @ 4:55 PM 5 comments

Where has Miss Science been?

Miss Science has been very busy working. She apologizes for taking so long. Hopefully, she will be able to resume her usual schedule now.

posted by Ask Miss Science @ 7:52 PM 1 comments

Bleach and Ammonia

The question about soap reminded me of this one I've been wondering about. Bleach and ammonia are both common ingredients in household cleaners, and I know that they are toxic in combination. But what is the difference between them, and why would I choose one over the other to clean a bathtub or toilet or floor? Do they both kill bacteria? Viruses?

-- Posted by Nancy to Ask Miss Science at 7/28/2004 07:57:43 PM

Bleach and ammonia are both bases. That is, both solutions contain hydroxide ions (OH^-). There are other reasons we can call them bases, too, but I'll leave that somewhat advanced discussion out.

To a chemist, ammonia is the gas NH₃, but commonly "ammonia" means a solution of the gas NH₃ in water.

"Bleach" isn't usually used as a chemical term. The closest common chemical term is "bleaching agent," which means exactly what you'd expect it means. Common household bleach is a solution of chlorine gas in water.

Common solutions of ammonia contain hydroxide ions because the gas, NH₃, produces them when it dissolves. The chemical equation describing the result of dissolving ammonia (the gas) in water looks like this:

NH₃(aq) + H₂O(l) <----> NH₄⁺(aq) + OH^-(aq)


Notice the arrow in that equation. It means that these species are all in equilibrium with each other -- they are all present at once. In other words, when you dissolve NH₃ in water (H₂O), some of the NH₃ stays more or less like it was when it was a gas, except that it is now surrounded by water molecules. Some of the other NH₃'s, however, react with nearby water molecules, taking a hydrogen ion (H⁺) away from them and leaving a hydroxide ion (OH^-).


Bleach contains hydroxide ions because chlorine doesn't dissolve very well in water. You see, if you make the water very basic -- by adding lye (NaOH), for example -- the chlorine will dissolve pretty well. The equation looks like this:

Cl₂(g) +2 NaOH(aq) <----> NaClO(aq) + H₂O + NaCl

Notice the double arrow, again. Let's talk a little more about equilibrium.

Chemical equilibrium is in a delicate balance. If you do something to change the number of any of the species present, the rest of the species in the equilibrium will react to the change. They react in a way that tends to counteract whatever change you made.

For example, assume you do something that adds extra OH^- ions to the ammonia solution. Now, there are too many OH^- ions; so, the whole system will react to correct that. To correct the influx, some of the NH₄⁺ ions and OH^- ions will react backwards and form NH₃ and H₂O. In the bleach equation, adding extra hydroxide (OH^-) ions causes the forward reaction to occur, which keeps the chlorine gas (Cl₂) dissolved. So, bleach typically contains a great excess of hydroxide ions.

Some of you might see, based on this, one reason why bleach and ammonia should not be mixed. Adding bleach causes the ammonia's reaction to go backwards, pushing the reaction to the left and then pushing the ammonia (gas) out into the air. NH₃, by the way, is very unpleasant to breathe. In actuality, there are other reactions going on. Depending on the temperature and on the relative amounts of bleach and ammonia, you might get ammonia (the gas), chlorine gas or one of a class of foul compounds called chloramines.

So, now that it is certain that we should not mix the two, the next question is which to use. If your concern is germ killing, bleach is certainly the way to go. However, ammonia does a pretty decent job, too. If you would like further information on the subject, I suggest this website containing an EPA report on the effectiveness of various cleaners. On this site a number of common household cleaners are compared in terms of effectiveness of removing soil and eliminating microbes.

The information used in this post is widely available on the internet and in chemistry textbooks.

posted by Ask Miss Science @ 7:52 PM 1 comments

Putting Nothing in Context

Edward Bowers sent me a question from an unproduced script he wrote for the old sitcom Charles in Charge. The script was titled "When You're a sophomore." Here's his question, in context:

My Charles in Charge script opens with the kids doing some homework. They get to exponents, and the rule of thumb about tens to the power of whatever. An argument ensues when they get to ten to the zeroeth ("Zeroeth?"). Just where do they get off making that one instead of zero? Seems like no tens multiplied by nothing should be zero. :-)

BUDDY [as if loved ones had lost their lives over this issue]

O-oh, don't get me started.

This is another of those questions that I can answer although it is a little outside my training. A mathematician would likely give a more thorough or more precise answer, but this one is basically correct. If any mathematicians have comments, I would happily receive them, and I would ask that they accept my apologies for any lack of precision.

There are two issues here. The first is that zero and nothing are not exactly the same thing. The other has to do with context. I'll tackle them in that order.

In certain circumstances, zero and nothing can behave in ways that are equivalent, even indistinguishable. But, in other cases, they are very different. To more easily tell when they are different, substitute "not anything" for the word "nothing."

First, consider addition:


If I add zero to 5, I get 5.

If I do not add anything to 5, I get 5.

Now, consider multiplication:

If I multiply 5 by zero, I get zero.

If I do not multiply 5 by anything, I still have 5.

Note, especially, that in the case of multiplication "nothing" and "zero" have very different effects.

So, when you say 10⁰, you are saying that there are no tens to be multiplied. You are not saying to multiply by zero.

Now on to the subject of context.

What if there are no tens there at all? What is there? Nothing? That is certainly a reasonable response in some sense. But is it really practical to assume there is absolutely nothing? At the very least, "nothing" is a bit unwieldy in mathematical terms. To wit, it's hard to work out where "nothing" goes on a number line.

Even if you ignore that last paragraph, it seems unlikely that you have "nothing" if you are concerned about multiplying it by tens.

In other words, there must be some sort of context in which one is or is not multiplying tens; otherwise, what difference does it make?

Let's consider some contexts.

If I write 5 x 10², then I am multiplying 5 by ten and then by ten again. The answer is 500.

If I write 5 x 10¹, then I am multiplying 5 by ten. The answer is 50.

If I write 5 x 10⁰, then I am not multiplying 5 by any tens. The answer is 5.

This latter is equivalent to multiplying 5 by 1. It is not the same as multiplying 5 by 0.

So, when there isn't a context already defined, a default context of "1" is used ("1" is the "identity element" for multiplication-- remember that?). This means that when you say 10⁰, you are, by implication, saying 1 x 10⁰.

In fact, any number raised to the power of zero is equal to one for the same reason:

A x B⁰ = A

Here, A is not being multiplied by any B's. The answer is A.

posted by Ask Miss Science @ 5:36 PM 0 comments

Time Travel for Good Neighbors?

Anonymous writes:

Dear Miss Science,



Given the following--

Misner space can exhibit more than one type of vacuum state, wherein a closed timelike curve could exist. If so, quantum effects do not automatically enforce Hawking's "chronology protection" in every case. Thus, with sufficient warping of space-time, a timelike curve could be created, making time travel possible.



--How much gravitational force is needed to create such a warp, and would this render time travel a practical impossibility, i.e., as creating a black hole in your front parlor might wreak havoc on the surrounding space-time (and annoy, if not reduce to protoplasmic jelly, the neighbors)?

Oh my!



This question is, of course, far beyond the intended scope of this blog. And, although I well appreciate the various levels of humor imbedded therein, it is also a question for which I do not have an answer. I did, however, enjoy looking up the relevant terminology.



The following part of this description is a bit beyond my training, so I must beg forgiveness from any physicists and mathematicians who might read this for any imprecisions they might find.



For those readers not familiar with the concepts relevant to the question, a mathematical "space" is a sort of grid or map upon which mathematical descriptions are based.



For example, you are probably most familiar with Euclidean space. Euclidean space is often described using Cartesian coordinates (the x, y and z axes you've probably seen before). This page at Mathworld gives a more complete description of the mathematical concept of a space.



Misner space is constructed from Minkowski space, which is the space used by Einstein in his special theory of relativity. As an aside, during my web-surfing, I found this article that declares Minkowski space to be a "glorious non-entity," an assertion I found humorous. The authors of that paper might find the reciprocal system of some interest. This is a system within which one of the primary assumptions is that space is Euclidean and only Euclidean.



That being said, I will return to more familiar territory and offer my quasi-educated guess, which is most assuredly sullied by wishful thinking: I think it can be done without the unfortunate side effects described above.



The only other statement I have is a word of caution to those investigating such phenomena. It is prudent always to remember that mathematics is a language. It is a means for codifying and describing things real and imaginary. Whenever one uses a language, which is most of the time, one should keep in mind that the language one uses deeply influences the sorts of thoughts one might have. Benjamin Lee Whorf had much to say on the subject. The curious reader is encouraged to investigate a collection of his essays called Language, Thought and Reality. Whorf was a chemist, too, the dear man. It is for these sorts of reasons that I have stopped using phrases such as "this process is governed by such-and-such a mathematical relationship." Instead, I say the process is described by the relationship. I find it keeps me grounded. It can be easy to forget that nature does what nature does, not what the equations dictate.

posted by Ask Miss Science @ 11:52 PM 0 comments

Comments Fixed and Email Added

The issue with the comments appears to have been resolved.

Also, I added a visible email address.

posted by Ask Miss Science @ 5:16 PM 1 comments

How does soap work?

Alan says:

explain again how soap works

One of the main things soap does is to force water molecules to quit being so cliquish with each other.

You see, water molecules stick together something fierce. Much of their stickiness comes from the fact that each water molecule has an electric dipole. In other words, one end of the water molecule is positively charged and the other end is negative. The difference in charge is due to an unequal distribution of electrons in the molecule. You may have heard of this before if you ever heard water described as being a polar molecule.

Of course, as you know, water molecules don't keep completely to themselves; they do associate with certain other types of particles. Two examples are the ions in table salt and molecules of sugar.

However, dirt and grease aren't on water's "cool" list. Most dirt, oil and grease are made of molecules that do not possess significant electric dipoles. In other words, molecules in those substances are non-polar.

When water molecules come near these non-polar substances, they don't even consider unclustering. Perhaps you've seen water bead on a waxy surface? The reason it does that is because the water molecules stay crowded together as tightly as they can rather than interact with the waxy surface.

The net effect of all this is that, as you know, plain water doesn't do a very good job of cleaning grease and dirt off of things.

Enter soap.

Soap molecules have split personalities. On one end of a soap molecule, there is an electric dipole. Water molecules are very attracted to this end of the soap molecule. But, soap molecules also contain a long "tail" that has a structure much more similar to dirt or grease.

Once the soap molecules mix in with the water molecules, the long tails keep the water molecules from being able to crowd together so well. In technical terms, it is said that soap "reduces the surface tension" of water.

Since the water molecules cannot cluster together so tightly, they are more likely to slip into crevaces in the dirt or grime. Also, the long soap tails, not being so snobbish, can attract the grime via a much weaker, but still electrostatic, interaction called "London" or "van der Waals."

With the water and soap molecules now mixed in with the dirt, a little
agitation, such as rubbing your hands together, can loosen the dirt so that it can be rinsed away.

posted by Ask Miss Science @ 11:46 PM 14 comments

What's up with adding comments?

I've told Blogger about this...

I set the comments to do these things:

[1] Allow anonymous posts

[2] Send me an email when a comment is posted

So far, it does that. But, the comment doesn't show up on the blog. I'll copy comments into the questions, as usual.

Right now, I have two questions in queue, in case you're curious. Feel free to send more.

posted by Ask Miss Science @ 12:03 AM 2 comments